There are materials having another special property: their refraction index is different for left and right circularly polarized light. This phenomenon is called circular birefringence.
Any linearly polarized light can be obtained as the superposition of a left circularly polarized and a right circularly polarized light wave. Therefore, if linearly polarized light traverses a medium that shows circular birefringence, its properties will change because the the two circularly polarized components slow down in the medium to a different extent.
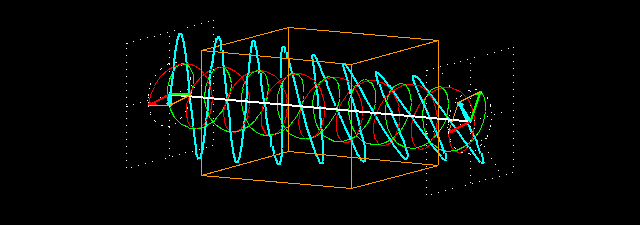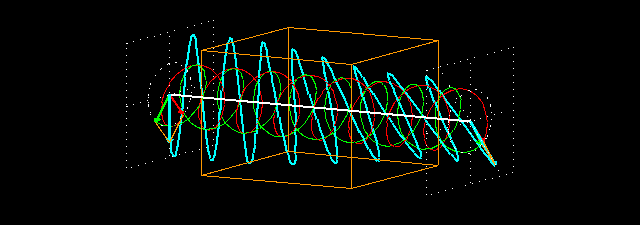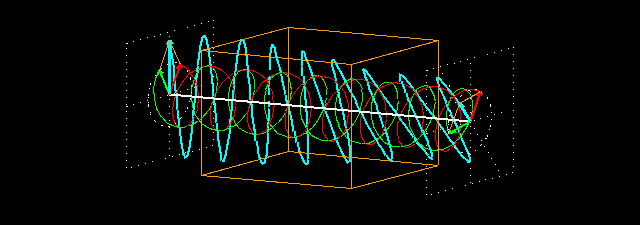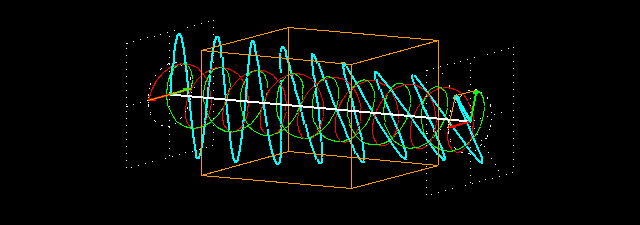
The following animation shows what happens when a plane-polarized (the plane of polarization is vertical here) light wave (indicated by light blue color) traverses a medium that does not slow down the left circularly polarized component (this is the circular wave shown in red) of the wave at all but slows down the right circularly polarized component (this is the circular wave shown in green) somewhat. For the latter component, the refraction index of the material is n=1.05.

We placed an intersecting plane before and after the piece of material and we show the field vectors at the point of intersection of the light beam and the intersecting planes. The following animation shows the intersecting planes from the front. On the left, the plane before the material; on the right, the plane after the material.
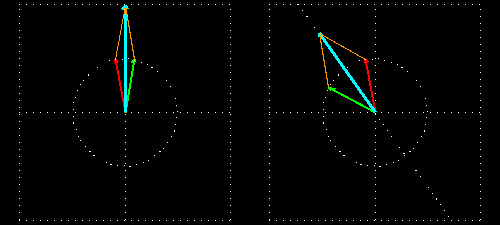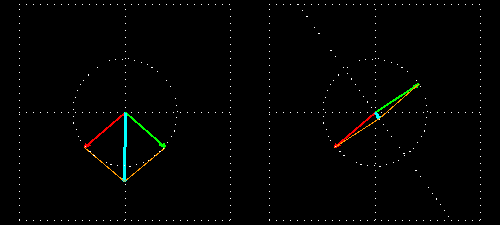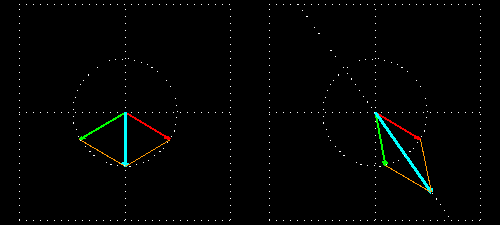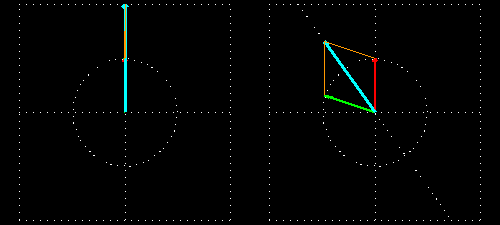
As you can see, the exiting wave continues to be a plane-polarized wave but its plane of polarization is no longer vertical; the polarization plane has rotated by 36 degrees.
What is the reason for that? The component shown in red traverses the medium unchanged while the component shown in green gets slower and its wavelength decreases in the medium. This slowdown and the decreased wavelength is hard to see in the figure because the refraction index (1.05) is close to 1.0 (we did not use a greater refraction index here because that would result in a confusing image). But as a result of this refraction index, the wave shown in green makes 4.2 full periods in the medium instead of 4 full periods, therefore its field vector will be 72° further on its rotating path than if the medium were not present.
And what happens to the sum of the two circular components? Before the medium, the field vectors of the components coincide when they point vertically up or down. But after the light exits the medium, this situation changes. When the red component points vertically up, the green component makes a 72-degree angle with the vertical direction. Their vectorial sum slants 36 degrees from the vertical direction. The superposition of the two circularly polarized components is a plane-polarized wave with a plane of polarization that is rotated by 36° with respect to the original polarization plane.
Of course, it is very unusual for a material to have a refraction index of 1.0 for one circularly polarized component. Real materials usually have refraction indices greater than 1.0 (but not equal) for both circular components. It was only for the sake of simplicity that we presented a situation where one component is not slowed down by the material at all.
The angle by which the polarization plane of the light exiting the medium rotates with respect to the original polarization plane is determined by the difference between the refraction indices for the two circularly polarized components (and of course on the length of path traversed in the medium). If the difference between the refraction indices is large enough and the piece of material is thick enough then the plane of polarization can make several full rotations as light traverses the material.
Please note: circular birefringence rotates the plane of polarization of plane-polarized light.
Materials that rotate the polarization plane of the light traversing them (this is optical rotation) or make plane-polarized light elliptically polar are called optically active materials. As we can see, optical activity is caused by circular birefringence or circular dichroism.
Notice: The word "birefringence" means double refraction (bi-refringence). If the adjective "circular" does not precede it then it refers to those materials (crystals) whose refraction index depends on the direction of the light.